Common Transversals and Tangents Frank Sottile University of Masssachusetts, Amherst Symbolic Computational Algebra 2002 University of Western Ontario

    We describe some interesting symbolic computations that arose while studying the following simple geometric problem:

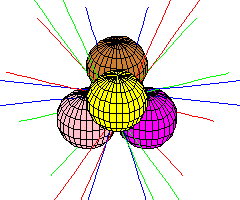
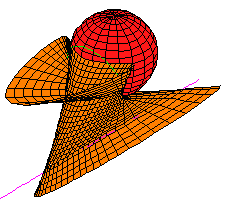
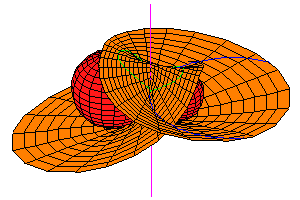
    Consider k lines and 4-k spheres in R³.

1. When the given lines and spheres are general, how many lines are there that meet the fixed lines and are tangent to the spheres. Call these common tangents and transversals.
2. For which arrangements of lines and spheres are there infinitely many cmmon tangents and transversals?

    Besides the interesting geometry, computation, and pictures, we feel that the study of geometry in 3-space is a fertile area for potential applications of symbolic and computational algebra. The results in this talk represent joint work with Gabor Megyesi and Thorsten Theobald.
    A web page featuring many, many pictures from this study.