 |
Frank Sottile, Texas A&M University | ||
|
Friday, 21 September,
Sam Houston State University |
|||
|
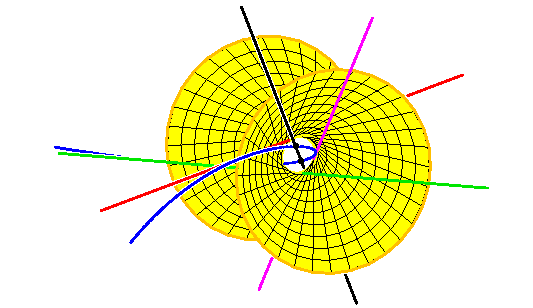
About 12 years ago, Boris Shapiro and Michael Shapiro made a remarkable conjecture about
real solutions to geometric problems coming from the classical Schubert calculus
on a Grassmannian.
The conjecture was proven recently by Mukhin, Tarasov, and Varchenko, using a deep
connection between integral systems and Schubert calculus.
This was popularized through extensive computational evidence,
and these computational experiments led to a subtle extension of it to flag manifolds.
A special case of this generalization was proven by Eremenko and others, and their
work suggests a generalization of the original Shapiro conjecture.
A feature of this story is an interesting dialog between theory and experiment.
In my talk, I will introduce the Shapiro conjecture and some of its extensions. In particular, I will describe the large-scale experiments we have run to formulate these extensions and the convincing evidence found. This is joint work with Luis Garcia, and our research team at Texas A&M University. |
|||
|
|
||