No Ramification ? 14
For the case of the Shaprio Conjecture pertaining to rational functions, Eremenko and Gabrielov have a new and elementary proof whose main ingredient that the Wronski map
W   :   Gr(2, m+2)   ---->   P2m ,
is unrammified over the set of polynomials of degree 2m with 2m distinct real roots.

    We expect ramification of the Wronski map over polynomials with multiple roots. Other ramification points are polynomials F(t) of degree mp with distinct roots s1, s2, ..., smp  where the system of equations

(*) det
G(si )
H
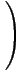 =  0 for each i = 1,...,mp
on points H of the Grassmannian has a solution with multiplicity.

    The asymptotic result mentioned previously showed that when the roots si  are sufficiently clustered, then there are no multiple solutions to the equations (*).

    In the experimentation, no multiple solutions were encountered in any instance of these equations (*) or in the equations for general incidence conditions, when all roots si were real.