Shapiro Conjecture : Transversality Conjecture

Transversality Conjecture

Transversality Conjecture
If the points s_i are real and distinct, then there are no multiple solutions to the equations

(*)

det

G(s_i )

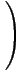= 0

for each i = 1,...,mp

or to the equations for general incidence conditions.

If the Transversality Conjecture holds, we can analytically follow the solutions from those constructed in the asymptotic result, and this implies the full Shapiro Conjecture.

The discriminant is a polynomial in the parameters s₁, s₂, ..., s_mp whose zeroes are exactly the values of the parameters giving multiple solutions, which also correspond to polynomials F(t) over which the Wronski map is ramified.

The Transversality Conjecture implies that the discriminant does not vanish when the parameters s₁, s₂, ..., s_mp are real and distinct.