5.14 Maximally Inflected Quintics With a Box Point

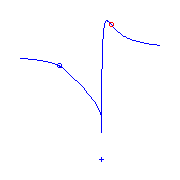
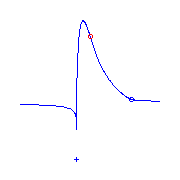
    This file displays maximally inflected quintics with a box point, which is a point of ramification of order 4. The intent is to display one example of all such curves, but there a quite a few of these curves, because there are many possibilities for the remaining ramification, and for its placement. The table below summarizes the possible types of ramification that can occur in such a curve, and our symbol for each type. The linked indices are to the spot in this file where curves with at most that that ramification are displayed.

Name Index Partition Germ Symbol Flex (0,1,3) 1 (s,s3) Red Circle Cusp (0,2,3) 11 (s2,s3)   Planar (0,1,4) 2 (s,s4) Green Circle Claw (0,2,4) 21 (s2,s4)   Flat Flex (0,1,5) 3 (s,s5) Magenta Circle Beak (0,2,5) 31 (s2,s5)

A Box Point