Some pictures of implicitly defined functions.

Here are some pictures of implicitly functions. For most, I have included the polynomial that defines the curve. The last 2 (in blue) are classically important curves. (See below)

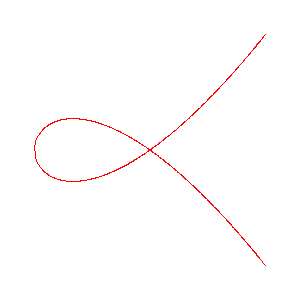
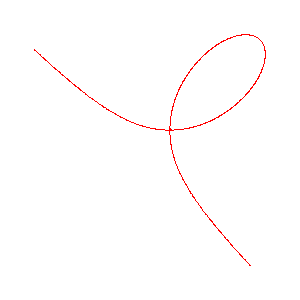
x3+y3-4xy	y2-x4+x2
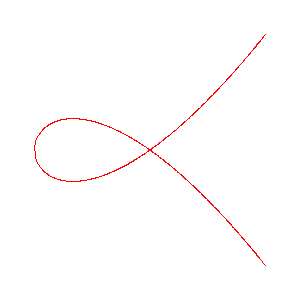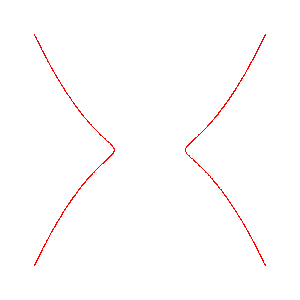
y2-(x3+3x2)	y(y2-1)(y-2) -x(x-1)(x-2)
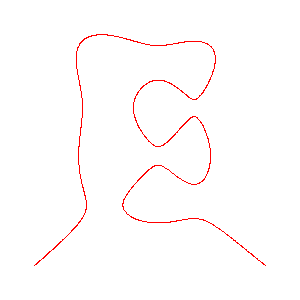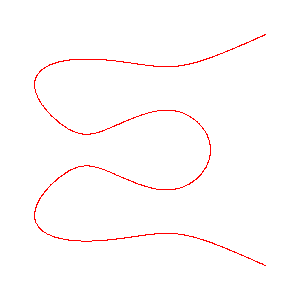
-y(y2-1)(y2-4) -x(x-1)(x2-4)-1/5	2y3+y2-y5 -x4+2x3-x2
	(x2 + 2y2-2)(2x2 + y2-2)+ 1/20

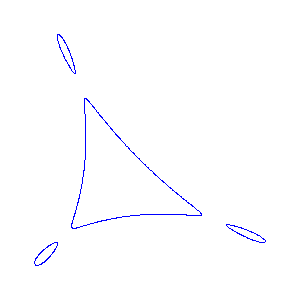
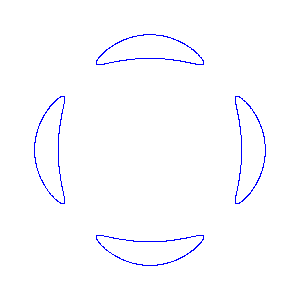
The blue curves are classically important. The one of the left was constructed by Harnack in 1876, and the one on the right by Hilbert around 1900. Both are examples of degree 4 curves with 4 ovals, the maximum possible number of ovals (This fact is due to Harnack). The curves are quite different.