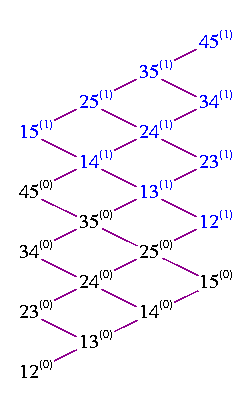
The quantum Bruhat order for degree 1 curves
in the Grassmannian of 2-planes in 5-space.
|
COCOA VI Villa Gualino Torino, Italia 3--5 June 1999 Frank Sottile University of Wisconsin-Madison |
The maximal minors of a generic rectangular matrix satisfy interesting quadratic relations. A reduced Gröbner basis for the Plücker ideal of these relations is elegantly described in terms of a natural Bruhat order defined on the set of maximal minors. This shows the maximal minors generate an algebra with straightening law on this Bruhat order, and these results are classical.
If we now consider a generic matrix of polynomials in a variable t, then the maximal minors are themselves polynomials in t. The goal of this talk is to describe a reduced Gröbner basis for the ideal of algebraic relations among these coefficients. This will show that the coordinate ring of the quantum Grassmannian (a singular compactification of the space of rational curves in the Grassmannian) is an algebra with straightening law on a quantum Bruhat order.
We will begin by reviewing the classical situation described above,
including some interesting geometric consequences of the reduced
Gröbner basis for the Plücker ideal. We will then describe a
reduced Gröbner basis for the quantum Plücker ideal, indicate our
method of proof, and describe some consequences of these relations. This
talk represents joint work with Bernd Sturmfels.