Shapiro Conjecture : Monotone Conjecture

Monotone Conjecture

The example of tangent and secant lines shows that we cannot expect partial flags satisfying incidence conditions imposed by osculating flags to be real; The Shapiro Conjecture fails for partial flags. Something can however be salvaged.

Suppose that we have Grassmannian conditions of index i₁ <= i₂ <= ... <= i_k.
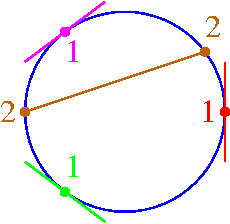
Points s₁ , s₂ , ... , s_k on the rational normal curve g are monotone if they occur in order along g.

Monotone Conjecture
All partial flags satisfying Grassmannian conditions imposed by flags osculating the rational normal curve at monotone choices of points will be real.


Monotone Solutions always real		Not Monotone Solutions not always real