More Restrictions for $ k=2$      
Theorem.
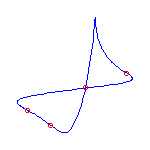
1)   A maximally inflected quintic with 4 cusps
      and 1 flex has either 1 or 2 ($ =g$) real nodes.

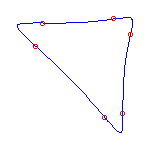
2)   A maximally inflected sextic with 6 cusps
      has either 3 or 4 ($ =g$) real nodes. 		 

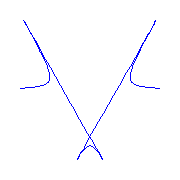
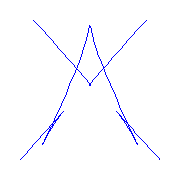
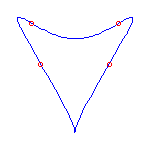
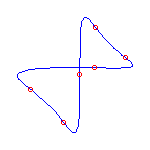
Proof:
1)   Such a curve is dual to a maximally inflected quartic with 1 cusp and 4 flexes.
2)   Such a curve is dual to a maximally inflected quartic with 6 flexes.
    These curves always have the geometry displayed below.