Line tangents to four triangles

H. Brönnimann, O. Devillers, S. Lazard, and F. Sottile
Read the translation into Swedish by Lera Domartina, or the translation into Spanish by the Ibidem Group or the translation into Croatian by Milica Novak.
We consider the following simple geometric question: What is the maximum number of lines that are tangent to four triangles? (That is, we are counting the lines which meet one edge from each triangle.) For simplicity, we assume that the triangles are in a suitable general position, in that the algebraic relaxation where we replace edges by supporting lines has only finitely many solutions. (In fact 162 distinct complex solutions.)

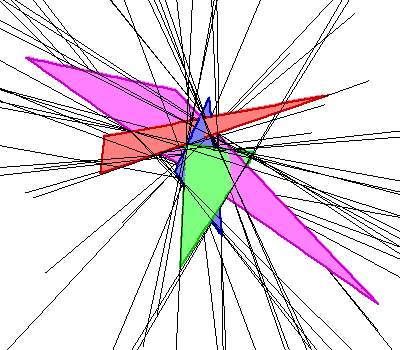
    We ask for the maximum possible number, because the minimum is zero: If the four triangles are sufficiently far apart, say at four corners of a very large room, then there will be no such common transversals.

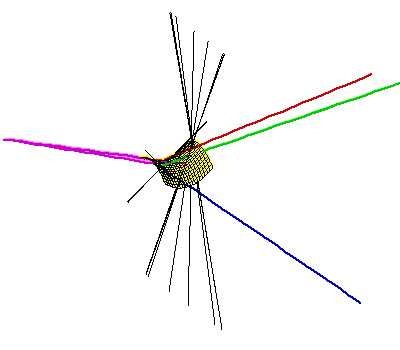
    Currently the best answer to this question is that the maximum number is between 62 and 162, with an upper bound of 156 if the triangles are disjoint. The upper bound is almost surely not the best possible, and we also doubt the optimality of the lower bound. This lower bound is due to a construction that we describe in this page (linked to the picture below on the right). This construction involves perturbing four line segments having 2 common transversals, and each of the resulting triangles have one extremely small angle - they are quite thin. Our best construction involving four fat triangles has 40 common transversals. A description of the computer search we used to find this example is linked to the picture on the left below, as well as animations.

    This WWW page accompanies our article on this subject, On the number of line tangents to four triangles in three-dimensional space.
The pictures are linked to further discussion

       
Animations: 841 kB   2100 kB   4198 kB.         Animations: 86 kB   215 kB. 526 kB.
Based upon work supported by the National Science Foundation under CAREER Grant DMS-0134860.
Written on: 30 December 2004 by Frank Sottile
Last modified: Mon Apr 1 12:28:59 MDT 2019