Previous: 5.2 Quintics with 7 flexes and
1 cusp
Next: 5.4 Quintics with 5 flexes and 2
cusps
Up: 5. Maximally Inflected
Quintics
5.3 Maximally Inflected Quintics With 7 Flexes and one Planar point
Here, we draw all the rational plane quintics with 7 flexes at the points
3,-2,-1,0,1,2,3 and one planar point at infinity.
There are 21 such curves in all.
However, since the set of ramification points is fixed under the involution
that sends s to -s, we may reparameterize any such curve
(reversing the direction of parameterization) to obtain another.
This either gives the same parameterized curve back (up to fractional linear
transformation in the plane), or it gives a different curve.
The images of the original curve and the reparameterization, as
unparameterized curves in the plane are identical.
An artifact of the method we use for drawing such curves is that the
reparameterized curve is the image of the original curve, reflected about
some vertical axis.
Thus the curves which equal their reparameterization are symmetrical while
those which do not are asymmetrical.
Altogether, there are 3 symmetrical curves and 18 asymmetrical curves
with this choice of ramification.
(In fact, this decomposition of 3 and 18 persists for any similar symmetric
(under s maps to -s) choice of the 7 flexes with a planar point at
infinity.
Below, we display the 21 curves.
We mark the positions of the flexes by red circles.
Some curves have only 6 flexes displayed; the 7th flex is on the line at
infinity.
We mark the positions of the planar point by a
green circle.
We indicate the positions of the solitary points by
green crosses.
Some curves have a magenta line
those curves have a pair of complex nodes, and the
magenta line is the real line on which the complex
conjugate nodes lie.
While this magenta line appears to be tangent to
the curve, it is not. (If it were, then it would have intersection number 6
with the complex curve, a quintic, and that violates Bézout's Theorem.)
We strongly urge the reader to print out this page,
the page of quintics with 7 flexes and one cusp,
as well as the page consisting of quintics having
9 flexes and compare the pictures.
Lastly, the MAPLE files used to draw these pictures are found in the
subdirectory
7flexes1planar.maple/.
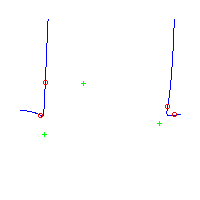
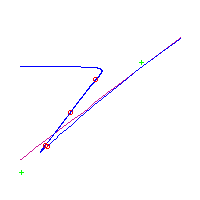
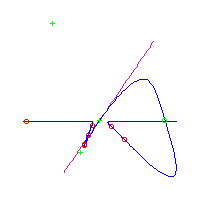
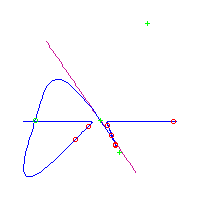
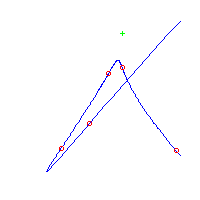
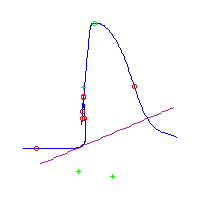
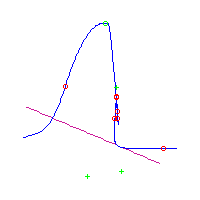
Curves with 3 solitary points
There are five curves with three solitary points.
The first curve is symmetric and has 3 nodes.
The remaining curves are in asymmetric pairs,
and each had one node and two comples nodex.
Each pair has a solitary point close to a branch
of the curve, and the pictures on the left are
a magnification of the interesting part of the
curves on the right. |
 |
 |
 |
 |
 |
 |
 |
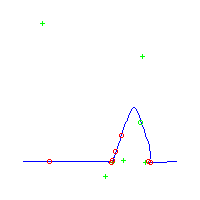
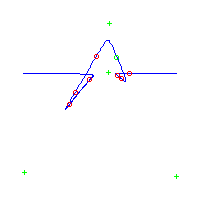
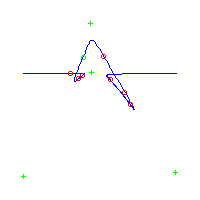
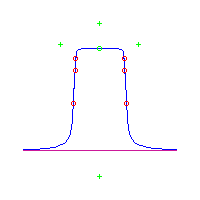
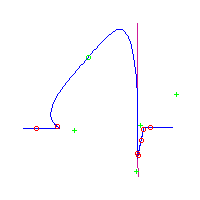
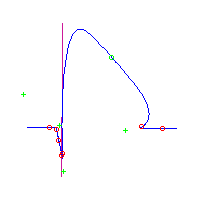
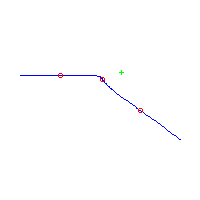
Curves with 4 solitary points
There are 9 curves
with 4 solitary points.
Four have two crossings,
while the rest have
none but do have a pair
of complex nodes.
|
 |
 |
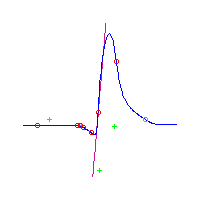
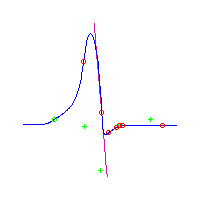
The first of the five
remaining is symmetric,
and the last asymmetric
pair has a solitary point
nestled close to a branch
of the curve and to three
flexes. We magnify and
display the interesting
part of one curve. |
 |
 |
 |
 |
 |
 |
 |
 |
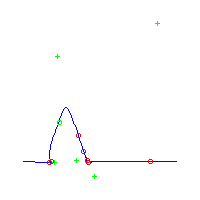
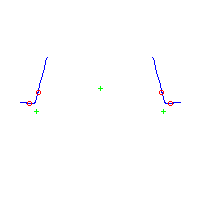
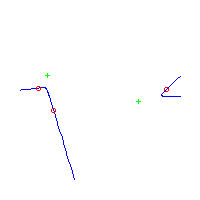
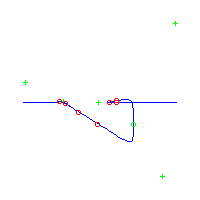
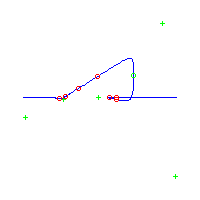
Curves with 5 solitary points
There are five curves
with 5 solitary points.
The first is symmetric,
and two of its solitary
points are obscured by
nearby flexes.
To the right, we display
that curve, and also a
magnification of its
interesting parts.
The last two curves also
have an obscured solitary
point, and we again
magnify the interesting
part of the curve. |
 |
 |
 |
 |
 |
 |
 |
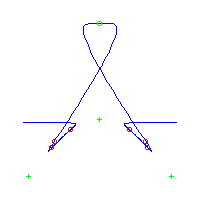
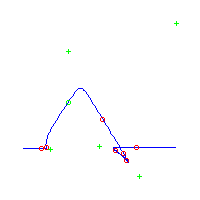
Curves with 6 solitary points
There are two curves (in one asymmetric pair) with 6 solitary points.
One of their solitary points is quite close a branch of the curve.
We magnify the interesting part of the curve and display it on the right.



Previous: 5.2 Quintics with 7 flexes and
1 cusp
Next: 5.4 Quintics with 5 flexes and 2
cusps
Up: 5. Maximally Inflected
Quintics