5.4 Pictures of Common Transversals and Tangents to a Symmetric Hyperboloid

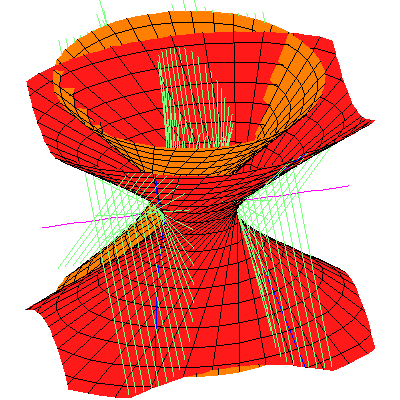
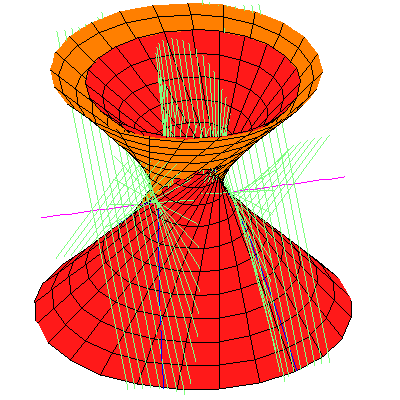
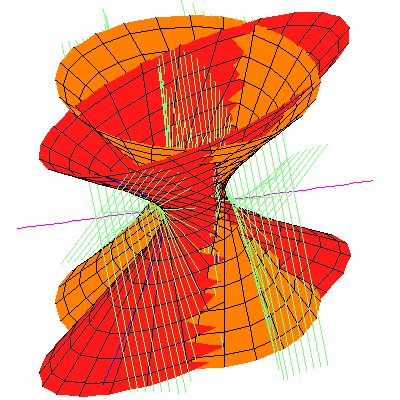
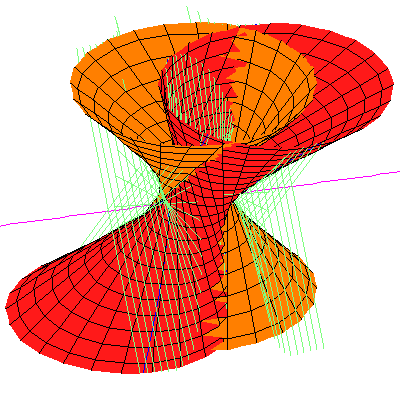
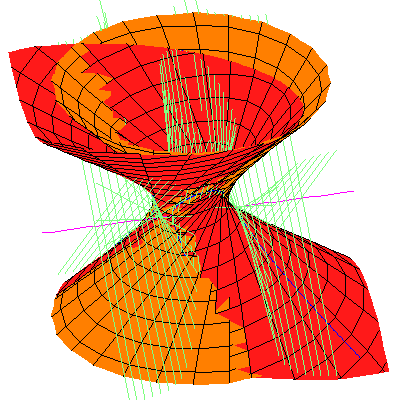
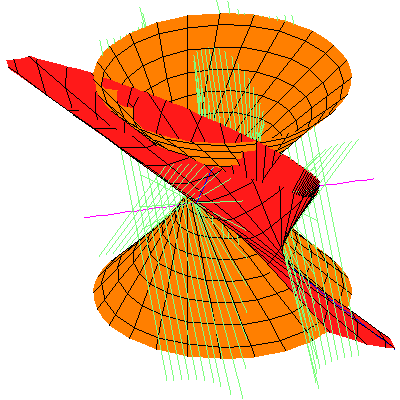
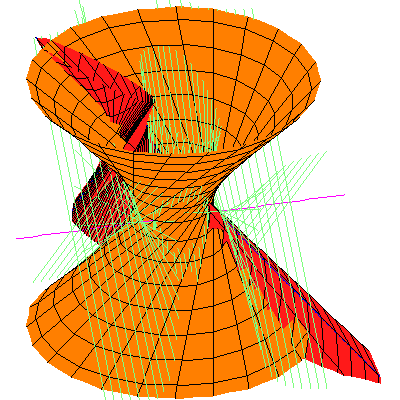
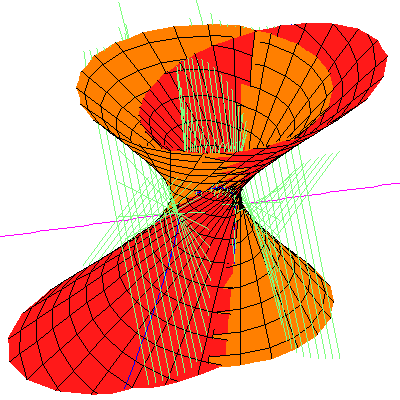
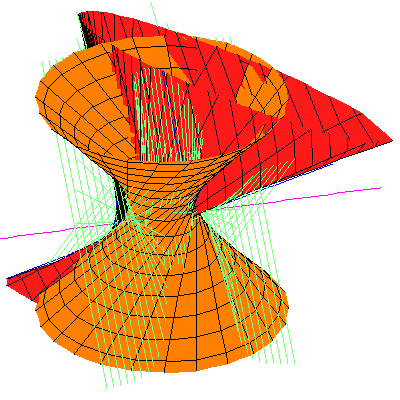
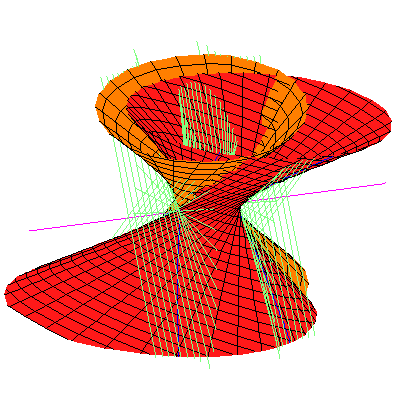
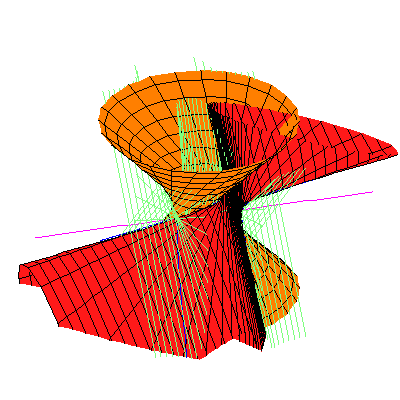
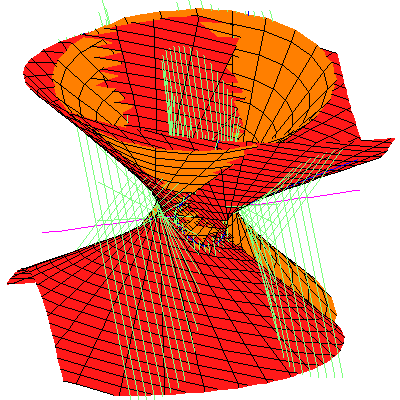
    In the pictures below, the hyperboloid in coral is the original hyperboloid and the lines perpendicular to the x-axis and tangent to this hyperboloid are drawn in light blue-green. Each quadric in red is a member of one of the families of quadrics having tangent to the same set of lines perpendicular to the x-axis as the original quadric. Here, unlike the other cases, all 12 families given by our Theorem contain real quadrics. We display one real quadric from each family. The links below each picture are to two maple files, and to an animation of the red quadric rotating. The first link is to the file that drew the picture, the second to the file that drew the rotating animation, and the third is the animation.

    Each family may perhaps best be thought of as parameterized by the points on a hyperbola with affine equation ab-1, with smooth quadrics corresponding to the points in the affine plane, but two singular members given by the two points at infinity. Our parameterization runs along one branch of this hyperbola, from a=sqrt(5)-2 to a=sqrt(5)+2, avoiding, but approaching the singular members.

    While we have not yet drawn animations of the families, the families may be visualized by considering the action of the non-zero real numbers, R^x, on R³ which acts by scaling the distance to the x-axis.