
CoAmoeba of the line t --> (t-1, t-ζ, t-ζ2),
where ζ is a primitive third root of 1.
More coAmoebae of lines in (C*)3.
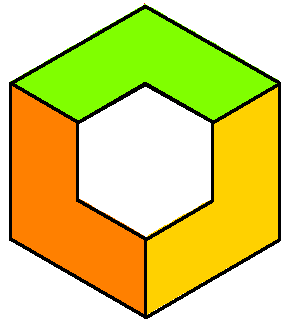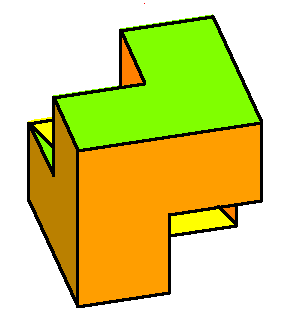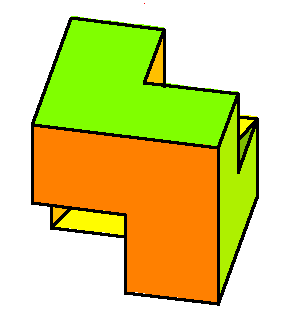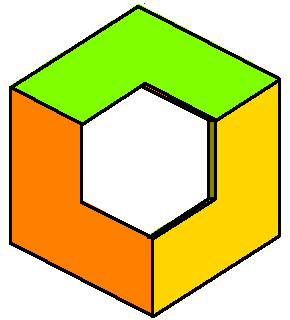
CoAmoeba of the plane x+y+z+1 =0 in (C*)3.
The coAmoeba of the plane and its phase-limit set.
The phase limit set of a varietyMounir Nisse and Frank Sottile |
|
A coamoeba is the image of a subvariety of a complex torus (C*)n under the argument map to the real torus (S1)n. We describe the structure of the boundary of the coamoeba of a variety, which we relate to its logarithmic limit set. Detailed examples of lines in three-dimensional space illustrate and motivate these results. |
|
Here are the coamoebae of a line and a plane in (C*)3.
The links are to further pictures.
|
|
 CoAmoeba of the line t --> (t-1, t-ζ, t-ζ2), where ζ is a primitive third root of 1. More coAmoebae of lines in (C*)3. |
 CoAmoeba of the plane x+y+z+1 =0 in (C*)3. The coAmoeba of the plane and its phase-limit set. |