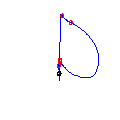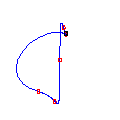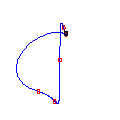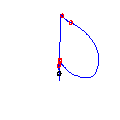
We introduce and begin the topological study of real rational plane curves,
all of whose inflection points are real.
The existence of such curves is a corollary of results in the real Schubert
calculus, and their study has
consequences for the important Shapiro and Shapiro
conjecture in the real Schubert calculus.
We establish restrictions on the number of real nodes of such curves
and construct curves realizing the extreme numbers of real nodes.
These constructions imply the existence of real solutions to
some problems in the Schubert calculus.
We conclude with a discussion of maximally inflected curves of low degree.