|
The necessary and sufficient Horn inequalities which determine
the non-vanishing
Littlewood-Richardson coefficients in the cohomology of a Grassmannian are
recursive in that they are naturally indexed by non-vanishing
Littlewood-Richardson coefficients on smaller Grassmannians.
We show how
non-vanishing in the Schubert calculus for cominuscule flag varieties
is similarly recursive.
For these varieties, the non-vanishing of products of Schubert classes is
controlled by the non-vanishing products on smaller cominuscule flag
varieties.
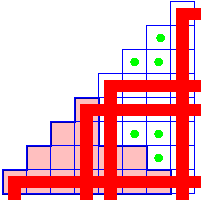
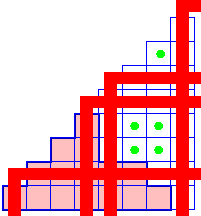
In particular, we show that the lists
of Schubert classes whose product is non-zero
naturally correspond to the integer points in
the feasibility polytope, which is defined by inequalities
coming from non-vanishing products of Schubert classes on smaller
cominuscule flag varieties.
While the Grassmannian is cominuscule, our necessary and sufficient
inequalities are different than the classical Horn inequalities.
|