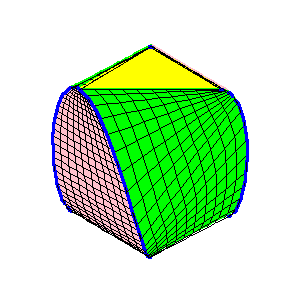
|
An orbitope is the convex hull of an orbit of a compact group acting
linearly on a vector space. These highly symmetric convex bodies lie
at the crossroads of several fields, in particular,
convex geometry, optimization, and algebraic geometry.
We present a self-contained theory of orbitopes with an
emphasis on orbitopes for the groups SO(n) and O(n). These include
Schur-Horn orbitopes, tautological orbitopes,
Carathéodory orbitopes, Veronese orbitopes and Grassmann orbitopes.
We study their face lattices, their algebraic
boundary hypersurfaces, and
representations as spectrahedra or projected spectrahedra.
|